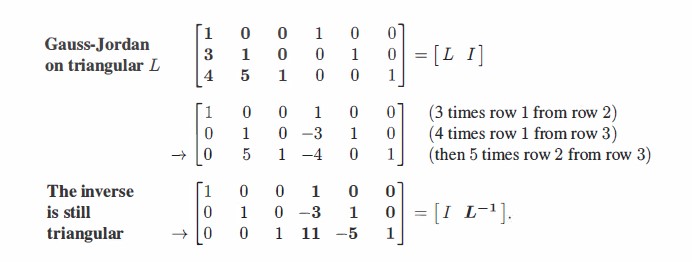本节介绍矩阵乘法,Gauss-Jordan法求逆阵。
一、矩阵乘法的四种方式
1、元素法
$$\begin{align}\begin{bmatrix}\cdots \end{bmatrix} \begin{bmatrix}\vdots \end{bmatrix}&=\begin{bmatrix} \cdot \end{bmatrix} \\
A \qquad B &= C
\end{align}
$$
\( Cij \) 例
\( \begin{align} C_{34}&=(row \; 3 \; of \; A)\cdot(column \; 4 \; of \; B)\\&=a_{31}b_{14}+a_{32}b_{24}+\ldots\ldots\\&=\sum_{k=1}^n a_{3k}b_{k4}\end{align} \)
2、列方法
$$\begin{align}\begin{bmatrix}\cdots \end{bmatrix} \begin{bmatrix}\vdots \vdots \end{bmatrix}&=\begin{bmatrix} \cdot \cdot \end{bmatrix} \\
A_{m \times n} \, B_{n \times p} &= C_{m \times P}
\end{align}
$$
\( C \) 中各列是 \( A \) 中各列的线性组合。
3、行方法
$$\begin{align}\begin{bmatrix}\cdots \\ \cdots \end{bmatrix} \begin{bmatrix}\vdots \, \vdots \end{bmatrix}&=\begin{bmatrix} \cdot \, \cdot \\ \cdot \, \cdot \end{bmatrix} \\
A_{m \times n} \, B_{n \times p} &= C_{m \times P}
\end{align}
$$
\( C\) 中各行是 \( B\) 中各行的线性组合。
4、列乘以行的方法
$$\begin{align} AB=Sum\;of\; (columns\;of\;A)\cdot(rows\;of\;B) \end{align}$$
\( AB\) 等于 \(A\) 的各列与 \(B\) 各行乘积之和。
例:
$$\begin{align}\begin{bmatrix}2&7 \\ 3&8\\4&9 \end{bmatrix} \begin{bmatrix}1&6\\0&0 \end{bmatrix}=\begin{bmatrix} 2\\3\\4\end{bmatrix} \begin{bmatrix} 1&6\end{bmatrix}+\begin{bmatrix} 7\\8\\9\end{bmatrix} \begin{bmatrix} 0&0\end{bmatrix}
\end{align}$$
另:矩阵的分块乘法(Block multiplication)
$$ \left[ \begin{array}{c|c} A_1 & A_2 \\ \hline A_3 & A_4 \end{array} \right] \left[ \begin{array}{c|c} B_1 & B_2 \\ \hline B_3 & B_4 \end{array} \right] =\left[ \begin{array}{c|c} A_1 B_1+A_2B_3 & \cdots \\ \hline \cdots & \cdots \end{array} \right] $$
二、Gauss-Jordan法求逆
方阵(square matrices)才可能存在逆阵,但不是所有方阵都有逆阵。
如果矩阵 \(A\) 可逆,则称矩阵 \(A\) 是可逆的(invertible)或称非奇异的(nonsingular),
其逆阵有:
$$ A^{-1}A=I=AA^{-1} $$
即左逆阵和右逆阵是相等的。
1.奇异矩阵的情况(singular case,no inverse)
例:
$$ A=\begin{bmatrix}1&3\\2&6 \end{bmatrix} $$
其行列式等于 \(0\)
关于奇异矩阵特征的另外一种表述:如果存在非零向量 \(X\) ,使得 \(AX=0\) ,那么矩阵 \(A\) 就是奇异的(或称不可逆的).
奇异矩阵,它的列是线性相关的,能通过线性组合得到 \(0\) .
2.非奇异矩阵的情况
例:
$$\begin{align}\begin{bmatrix}1&3\\2&7 \end{bmatrix} \begin{bmatrix}a&c\\b&d \end{bmatrix}&=\begin{bmatrix} 1&0\\0&1 \end{bmatrix} \\
A \qquad \qquad A^{-1} &= I
\end{align}
$$
求逆和解两个方程组是一回事
$$ A \times column \, j \, of \, A^{-1}=column \, j \, of \, I $$
这基本上回到了Guass消元法解方程组的问题。现在用“Guass-Jordan”方法考虑解决,此方法能同时处理多个方程组。
Gauss-Jordan 方法:
构建增广矩阵
$$ \left[ \begin{array} {cc|cc} 1&3&1&0\\2&7&0&1\end{array} \right] $$
用消元法,左侧 \(A\) 变为单位阵,同时右侧就得到了 \(A\) 的逆阵
$$ \left[ \begin{array} {cc|cc} 1&3&1&0\\2&7&0&1\end{array} \right] \to \left[ \begin{array} {cc|cc} 1&3&1&0\\0&1&-2&1\end{array} \right] \to \left[ \begin{array} {cc|cc} 1&0&7&-3\\0&1&-2&1\end{array} \right] $$
这可以用消元法的矩阵形式考虑
若 \(E\) 是消元的初等矩阵,则有
$$ E \begin{bmatrix} A&I \end{bmatrix}=\begin{bmatrix} I&A^{-1} \end{bmatrix} $$
即:$$ 若EA=I,则E=A^{-1},所以EI=A^{-1} $$